Finding Fifteen

Shall we play the game of Finding Fifteen? It is a fun elementary game, which also reveals a subtle game-theoretic concept when looked upon from a secret perspective.

Shall we play a game? Let us begin to explore the theory of games with several finite games that are fun to play and yet which will also illustrate a few subtle game-theoretic concepts.

Finding Fifteen

Consider first the simple game of Finding Fifteen. Two players take turns selecting numbers from 1 to 9, with each number selected at most once. Each player has the goal to have three numbers in their collection that sum to 15.

Perhaps you have selected the numbers 8, 2, 6, 1, for example, and you have won with the sum 8 + 6 + 1. Whoever first can form 15 by adding three of their numbers is the winner. Take care that it must be three numbers adding to 15, not two, so that while 8 + 6 + 1 is a winning sum, 8 + 7 is not. Repetition is not allowed in the winning sum, so neither 5 + 5 + 5 nor 4 + 4 + 7 count as winning. It might happen that neither player wins, if neither player can form 15 even after all nine numbers are chosen.

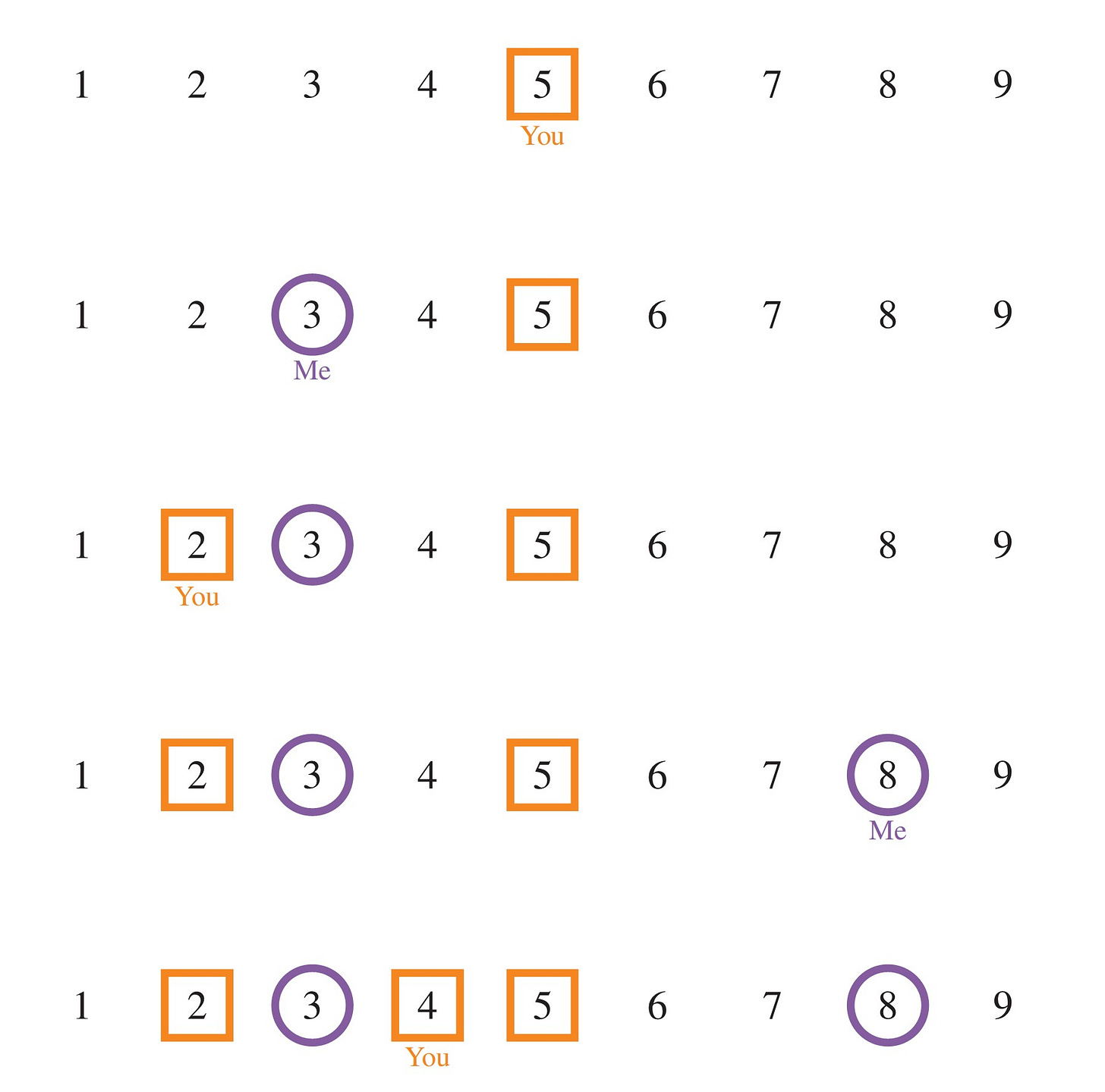
Let us suppose that our game proceeds with the following plays:

You: 5
Me: 3
You: 2
Me: 8
You: 4

Were those good moves? Were any of them forced? Who will win the game?

Interlude

We might represent the game history as below, where you have drawn squares around your selected numbers and I have used circles. In actual play, of course, we would simply play the game on one line without redrawing all the numbers. This is an easy way for us each to claim our numbers in turn.

Several of the moves in our game in fact were forced moves that had to be made just at that moment in order to avoid an immediate loss. For instance, after you played 2, I had to play 8 immediately to block your win via 5 + 2 + 8. But once I played 8, you had no immediately winning play, and so you had to play 4 to block my win via 3 + 8 + 4.

Now that you have selected the 8, I have no immediate winning move. What should I play? Do I have a good move?

Interlude

Shall we have a game?

Please enjoy this selection from Infinite Games: Frivolities of the Gods, my new book-in-progress serialized here on Infinitely More, with fresh material on games and the logic of games each week.

Follow along with my new class at Notre Dame on the philosophy and logic of games.

We begin with some fun finite games, which also happen to illustrate several subtle game-theoretic concepts.